Mathematicians are “reinventing the wheel” by giving it a new shape. Their newly imagined wheel looks like a many-dimensional guitar pick, and it could theoretically roll in ways beyond our three-dimensional understanding. This breakthrough solves a decades-old geometry problem by showing how to build objects in dimensions that we cannot envision .
“It’s a stunning theory,” says Gil Kalai, a professor at the Einstein Institute of Mathematics in Israel, who was not involved with the study. The results prove that these unfathomable objects can be constructed in any dimension at a fraction of the size of more traditional rolling shapes, such as circles or spheres.
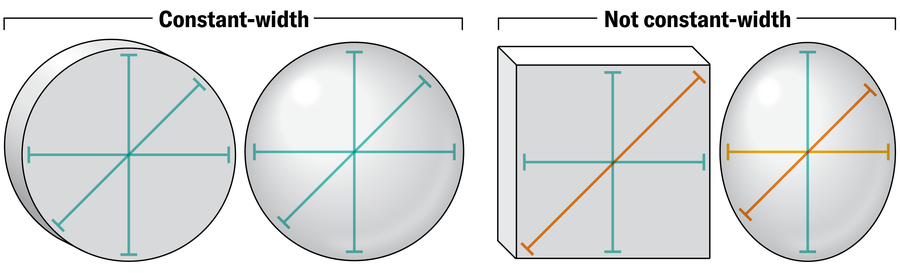
Wheels roll because they are objects with “constant width”—they appear to be the same width from every angle. This geometric property allows wheels to maintain a constant distance between two parallel planes, such as the ground and a car, as they move. Essentially, a shape has constant width if it can roll smoothly without wobbling. For example, place a tennis ball between your parallel hands and rotate it around—you’ll see that your hands never get closer together or farther away because the ball has constant-width geometry. An oblong shape such as an egg would fail that test.
On supporting science journalism
If you’re enjoying this article, consider supporting our award-winning journalism by subscribing. By purchasing a subscription you are helping to ensure the future of impactful stories about the discoveries and ideas shaping our world today.
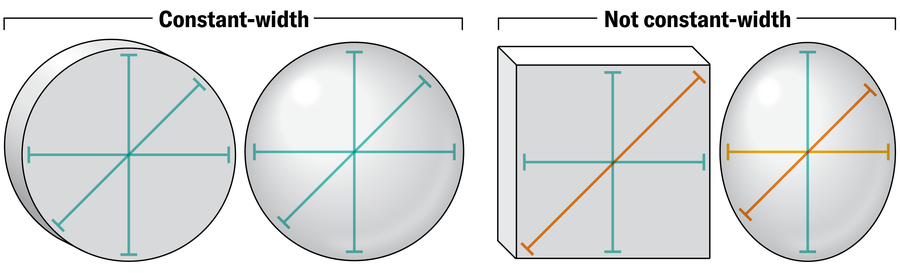
Circles and spheres are simple, intuitive examples of constant-width shapes, and humans have been using them to facilitate movement for millennia. These are special kinds of constant-width shapes called “balls”—shapes where all boundary points are the same distance from the center. This is a circle in two dimensions and a sphere in three, and the concept extends into higher dimensions that we can’t readily visualize.
Because the boundary points are all positioned at a fixed distance away from one central point, these balls are hefty: they have the maximum possible volume for a constant-width shape in any dimension. But being so voluminous isn’t always ideal. In the 1980s mathematician Oded Schramm posed the question: How can we find constant-width shapes with the minimum volume in any dimension? That “is a very basic question,” Kalai says, one mathematicians have been interested in solving ever since. “But nobody had any method of how to probe it.”
The problem remained until this June, when an international team of mathematicians proposed a new way to construct constant-width shapes. The researchers’ approach, involving the intersection of an infinite number of n-dimensional balls, was posted on the preprint server arXiv.org in a concise three-page proof. “The recipe itself is very simple,” says study co-author Andriy Bondarenko, a professor of mathematics at the Norwegian University of Science and Technology. Although using and analyzing this recipe is relatively straightforward, it took the researchers years to “[understand] why we should consider this recipe in the first place.”
Wheels in Flatland
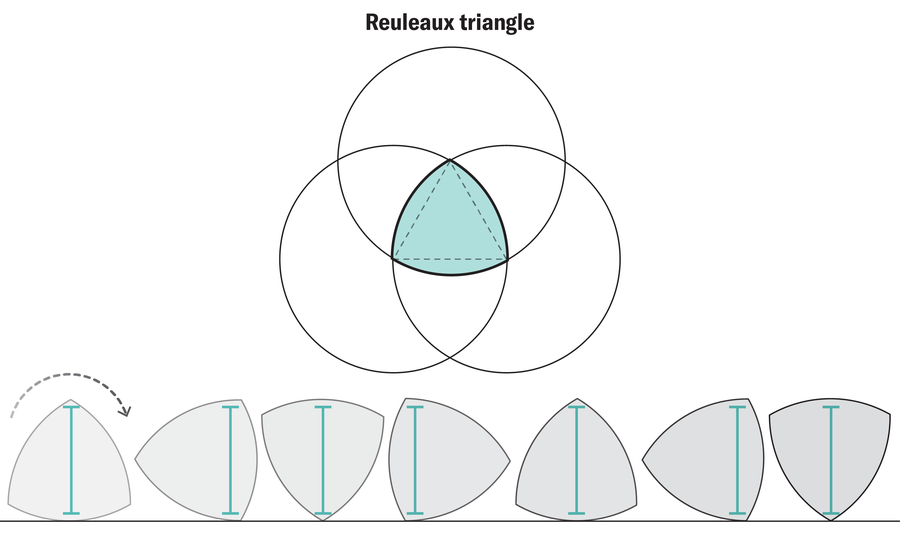
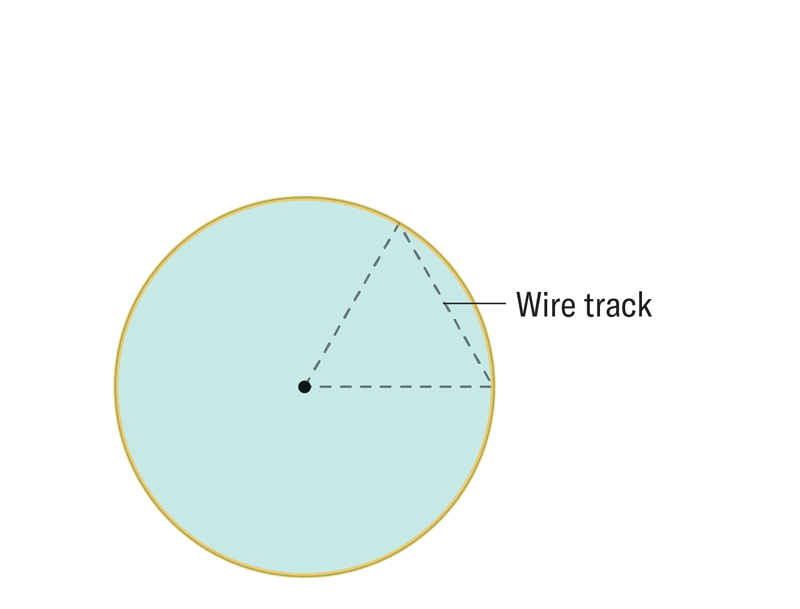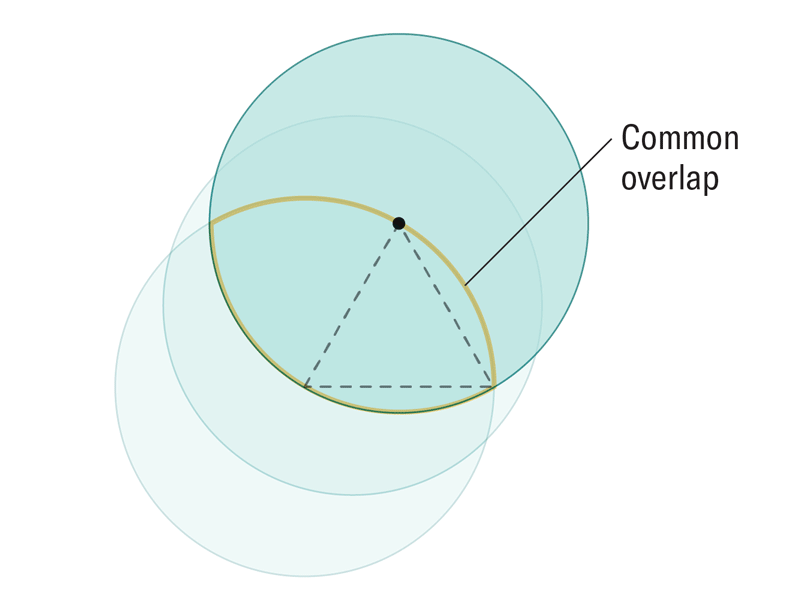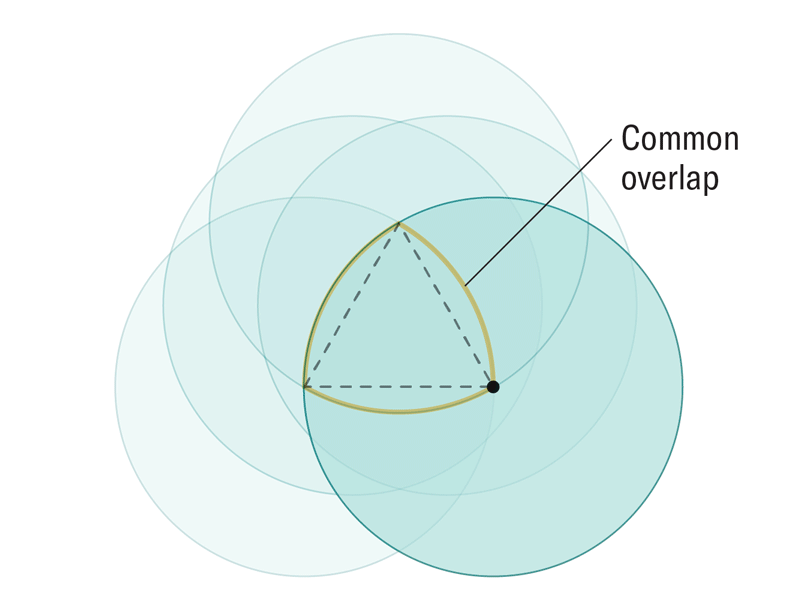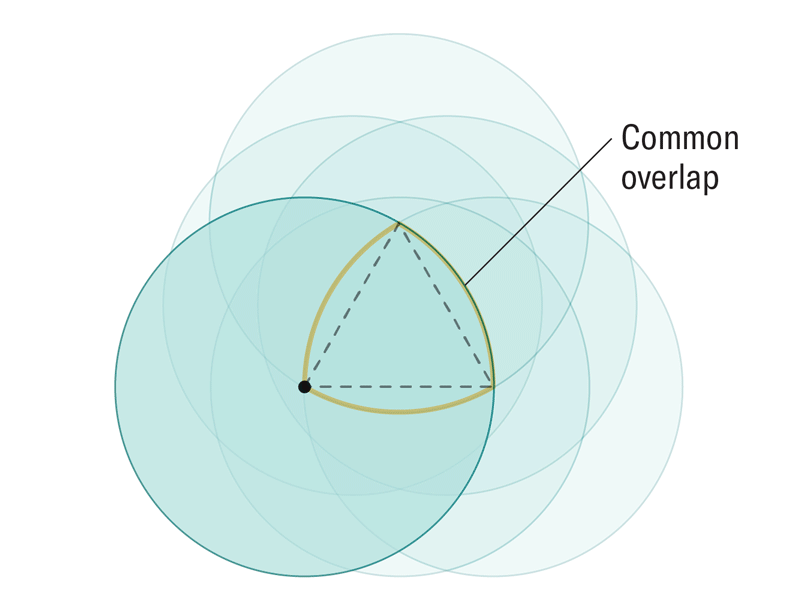
This latest work pioneers the investigation of constant-width shapes in any dimension, but designing wheels in two or three dimensions is itself not a new problem. For these easily understood lower dimensions, mathematicians have discovered many constant-width shapes with smaller volumes. In two dimensions, the Reuleaux triangle has the smallest area of constant-width shapes. You can draw this shape yourself using a sort of three-way Venn diagram. First, draw an equilateral triangle, then add three circles of equal radius around each corner. At the center of these circles, you’ll find a rounded shape that rolls like a circle with only a fraction of the size.
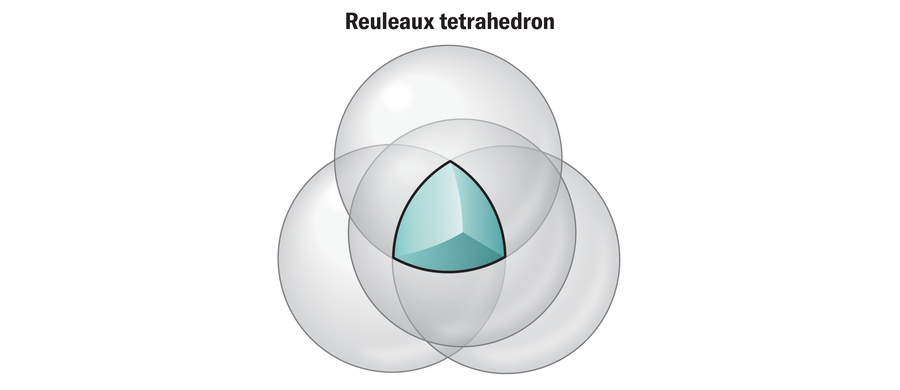
In three dimensions, you can use a similar method: start with a regular tetrahedron, a shape formed by four equilateral triangles, and add a sphere to each of its vertices. The resulting shape at the center of these overlapping spheres is known as the Reuleaux tetrahedron. It’s not exactly constant-width—it comes close, but the edges stick out too much. Some minor sanding down, however, gives rise to a constant-width shape. This can be done two different ways to form two slightly different shapes called Meissner bodies.
But the simple formulas behind these shapes offer no insights into how to build in four or more dimensions, which is beyond human perception. “It’s incredibly hard to generalize the Reuleaux construction,” Bondarenko says. “If it were easy, someone would have done it before.”
Wheels in Higher Dimensions
The latest work provides a general algorithm for constructing constant-width objects in any dimension by extending the Reuleaux intersection method. The team of mathematicians used a related Venn-diagram-like approach to yield the desired new shape—a geometrically anomalous nugget at the center of higher-dimensional space.
To represent this in two dimensions, again draw an equilateral triangle, followed by a circle centered around one of the triangle’s vertices, with a radius as long as each of the triangle’s legs. Then imagine moving that circle so that its center point follows the outline of the triangle, going up each leg and past each vertex before returning to where it started. As the circle moves, there are some places that it consistently occupies. This intersection of the infinitely many positions of the moving circle forms a familiar shape: the Reuleaux wheel, a generalization of the Reuleaux triangle. “By doing this, you essentially have the same construction as with the Reuleaux triangle, except here we take the intersection of infinitely many balls rather than just three or four,” explains Andrii Arman, a mathematician at the University of Manitoba and a co-author of the study.
This simple, computable technique can reveal an object of constant width in any dimension, so long as the boundary we drag our circle around is selected properly for each dimension. According to the new research, selecting this boundary in any dimension boils down to a basic recipe. In two dimensions, we trace the circle around a smaller quarter circle instead of an equilateral triangle. In three, we narrow this further from a fourth to an eighth of a sphere, and this pattern extends into higher dimensions by increasing powers of 2. Making such a boundary in n dimensions and moving a corresponding n-dimensional ball along it traces a higher-dimensional Venn diagram, the center of which the authors demonstrate must always have a width of exactly 2, yielding their new shape in any dimension.
This infinite, rather than finite, approach to building shapes not only ensures constant width but also makes computing their volume in higher-dimensional space straightforward. In comparison, prior constructions involve estimating an integral over many variables, while the latest work involves only two variables regardless of the shape’s dimension. “It’s really difficult to estimate volume in high dimensions,” Kalai says, yet “this whole [proof] is fairly simple and so elegant.”
The volume of the new object is 0.9n times smaller than an n-dimensional ball, which means that the volume decreases exponentially with each additional dimension. While the shapes decrease in size at an increasing rate when moving into higher-dimensional space, they are not the smallest possible objects that maintain constant width. “It’s conjectured that the Meissner bodies possess the smallest possible volume” in three dimensions, Bondarenko says, adding, “Our result is only 0.14 percent bigger than that.”
Kalai suggests that creating these shapes in higher dimensions with an infinite series “may be the dawn of [a new] era in the study of sets with constant width.” With the original problem now verified, “we are in uncharted territory,” he concludes, but armed with these new methods, “there is some hope to tackle many new problems.”